Mechanical Properties of Solids
A solid is defined by its size and shape. A force is necessary to change (or deform) the shape or size of a body. When you gently pull the ends of a helical spring, the length of the spring rises somewhat. When you remove the spring’s ends, it returns to its natural size and shape. Elasticity is a property of a body in which it tends to restore its original size and shape when the applied force is removed, and elastic deformation is the deformation produced by this property. When you apply force to a lump of putty or mud, however, it has little chance of regaining its original shape, and it becomes permanently distorted.
In engineering design, the elastic behaviour of materials is critical. For example, while designing a building, understanding the elastic properties of materials such as steel, concrete, and others is critical. The same may be said for bridges, autos, ropeways, and other structures.
Elasticity
When the forces that caused the deformation are eliminated, elasticity refers to the ability of a distorted material body to return to its original shape and size. Elastic behaviour (or response) is a term used to describe a body that has this flexibility.
Most solid materials exhibit elastic behaviour to some degree, but the size of the force and the resulting deformation within which elastic recovery is possible for any specific material is limited. This limit, known as the elastic limit, is the greatest stress or force per unit area that can occur within a solid material before irreversible deformation occurs.
Consequences of Elastic Limit
Most solid materials exhibit elastic behaviour to some degree, but the size of the force and the resulting deformation within which elastic recovery is possible for any specific material is limited. This limit, known as the elastic limit, is the greatest stress or force per unit area that can occur within a solid material before irreversible deformation occurs.
The elastic limit varies greatly depending on the type of solid being evaluated; for example, a steel bar or wire may only be stretched by roughly 1% of its original length, whereas strips of certain rubberlike materials can be stretched by up to 1,000%. Steel, on the other hand, is significantly stronger than rubber because the tensile force necessary to achieve maximum elastic extension in rubber is far lower (by a factor of roughly 0.01) than that required in steel. Many solids in tension have elastic characteristics that fall between these two extremes.
Elastic Behaviour of Solids
Any solid substance that is subjected to an external force is deformed, and the atoms or molecules that make up the body are shifted from their original positions, disrupting the condition of equilibrium.
The displacement causes the fixed points to vary, resulting in changes in interatomic and intramolecular distances. As a result, deformation can be defined as a change in the structure of any object caused by the action of a force. The deforming force is the force that causes the shift in the displacement of these particles.
We know that every force has an equal and opposite force operating against it. The restorative force, which acts in the opposite direction, opposes the deforming force. When the deforming force is removed, this force forces the body back to its original position.
The spring ball system is made up of balls that represent atoms and springs that represent the forces that act between them.
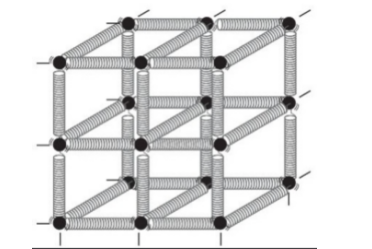
Stress and Strain
Stress:
Stress is defined as the force applied to a material divided by the cross-sectional area of the substance.
∂=F/A0
∂=stress
F=force
A0=initial cross-sectional area
Strain:
Strain is the material’s deformation or displacement as a result of applied stress.
ξ=Ln-L0/L0
ξ=strain
Ln=new length
L0=initial length
Hooke’s Law
Hooke’s law is a law of elasticity developed by the English scientist Robert Hooke in 1660, which states that for relatively mild deformations of an object, the displacement or amount of a deformation is precisely proportional to the deforming force or load. When the load is removed under these conditions, the item returns to its original shape and dimensions. The fact that minor displacements of their constituent molecules, atoms, or ions from normal locations are proportional to the force that generates the displacement explains the elastic behaviour of solids according to Hooke’s equation.
Stretching, compressing, squeezing, bending, and twisting can all be used to distort a solid. According to Hooke’s law, a metal wire exhibits elastic behaviour when stretched by an applied force since the modest increase in its length doubles each time the force is doubled. Hooke’s law asserts that the applied force F equals the displacement or change in length x times a constant k, or F = kx. The value of k is determined not only by the type of elastic material but also by its dimensions and shape.
Formula of Hooke’s Law
As long as the load does not exceed the material’s elastic limit, many materials will obey this law of elasticity. Linear-elastic or “Hookean” materials are those for which Hooke’s law is a useful approximation. Hooke’s law states that stress is proportional to strain in a direct relation.
Hooke’s law is expressed mathematically as:
F=−kx
Here,
x is the displacement of the spring’s end from its equilibrium position
F is the restoring force exerted by the spring on that end
k is a constant called the rate or spring constant
Elastic Modulus
We know that when an elastic material is distorted by an external force, it maintains an internal resistance to resist the deformation and returns to its original state once the external force is removed.
The various types of elastic moduli are as follows:
- Young’s Modulus: Young’s modulus is a property of an object that allows it to endure changes in its length when longitudinal tension or forces, such as compression, are applied. The longitudinal stress divided by the object’s strain equals the Young’s modulus. The letter Y symbolises Young’s Modulus.
- Shear Modulus: The shear modulus, also known as the Modulus of Rigidity, is the ratio of shearing stress to shearing strain. The letter ‘G’ symbolises Shear Modulus.
- Bulk Modulus: The ratio of hydraulic stress to associated hydraulic strain is known as bulk modulus. The letter ‘B’ symbolises Bulk Modulus.
Conclusion
Experiments can be used to determine the relationship between stress and strain for a specific material under tensile tension. A test cylinder or wire is stretched by an applied force in a conventional tensile property test. The applied force required to create the strain, as well as the fractional change in length (the strain), are both recorded.
The applied force is gradually raised in steps, with the length of each step being recorded. The stress (which is the same magnitude as the applied force per unit area) and the strain produced are represented on a graph.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out



