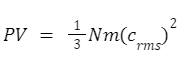Kinetic theory is a theoretical model that describes the molecular composition of gases concerning the evaluation of a large volume of submicroscopic particles such as atoms and molecules. This theory preaches that gas pressure tends to increase when particles collide with each other within a wall of a container. Kinetic theory of gases also defines properties of the gases such as thermal conductivity, mass diffusivity, and viscosity. Overall this theory provides a basic explanation of all the properties of gases related to the microscopic phenomenon. This theory is deemed significant because it helps in the development of a correlation between macroscopic properties and microscopic phenomena. In other words, kinetic theory helps in understanding and evaluating the action of molecules of gases that are consistently in motion. Due to consistent motion, molecules tend to collide with each other as well as with the walls of the containers in which they are placed or stored.

Assumptions for Kinetic Theory of Gases

Kinetic theory of gases tends to consider atoms and molecules of any gas as consistently moving masses that have huge inter-particle distances and could undergo elastic collisions. Implications of these assumptions are as follows:

1. Particles: Gas should be considered as a large collection of atoms or molecules.
2. Point masses: Atoms or molecules that form gas are microscopic particles.
3. The volume of Particles is negligible: Particles are often far apart from each other due to the presence of high inter-particle distance. Additionally, the inter-particle distance is comparatively larger than the size of the particle, due to which, there is a large, free and unoccupied space in the container. In this regard, the volume of the particle is negligible in comparison to the volume of the container.
4. Nil force of interaction: Particles are independent; thus, they neither have attractive interaction nor repulsive interaction with each other.
5. Particles are always in motion: Particles are consistently in constant motion due to the lack of interactions among them and because of ample availability of free space. Concerning this context, particles tend to move randomly in all directions rather than flowing in a straight line.
6. The volume of gas: Due to the constant motion, gas particles tend to occupy the total volume of the container irrespective of its size; hence, the volume of gas is considered to be equal to the volume of the container.
7. Mean free path: It is the average distance that a particle travels for meeting another particle independently.
8. Kinetic energy of particles: As the particles are in constant motion, they tend to have kinetic energy, which is proportional to the temperature of the gas.
9. The constancy of the momentum and energy: Moving particles tend to collide with each other as well as with the walls of the containers; however, the collisions are perfectly elastic. Due to this reason, collisions do not induce changes in the momentum and energy of the particles.
10. The pressure of gas: The collision of particles in a container exerts a force on the walls of that container and it is well established that force per unit of area is the pressure. In this regard, the pressure of the gas is directly proportional to the number of particles colliding in a specific unit of time and specific unit area.

Postulations of the Kinetic Theory of Gases

The kinetic theory tends to function on the following postulations for an adequate understanding of the macroscopic properties of gases:

1. Gases tend to comprise a large volume of tiny molecules and atoms. These particles are very small in comparison to the distance between the particles. The size of the individual particle is negligible due to which, most of the volume is occupied by the gas in space.
2. Molecules of the gas are in constant and random motion due to which they tend to collide with each other as well as with the walls of the container. Due to this collision, molecules impart momentum to the walls which result in the production of force. This force can be measured along with the pressure laid on the specific area of the container.
3. Collisions occurring between the molecules and the walls are perfectly elastic which means that molecules do not lose their kinetic energy even when they collide. Additionally, the molecules do not lose their speed despite the collisions.
4. The average kinetic energy of the gas particles tends to change with temperature which means that the average kinetic energy of the gas increases and decreases with an increase and decrease in the temperature respectively.
5. Molecules do not exert any sort of force of attraction or force of repulsion with each other except when they collide.

Kinetic gas equation

Where P denotes the gas’s pressure.

V is the volume of the gas

m is the mass of each gas molecule.

N is the total number of gas molecules in the volume V.

 crms is the gas’s root mean square speed.

Conclusion:

Overall Kinetic theory of gases focuses on considering the gas molecules as particles so that their movement and volume can be calculated. As per this theory, the pressure on gas molecules increases when they collide with each other or the surface of a container. Using this theory, multiple properties of gases are evaluated and determined.