According to the original function f, the domain of f is the range of the inverse function f-1. The range of f-1 becomes the domain of f. While plotting, (x, y) is swapped for (y, x) with the line y = x in mind. A function takes in a set of values, performs actions on them, and returns a result. When the inverse function is used, it agrees with the result, works, and returns to the original position. Continue reading to learn more about the inverse function, how to find it, and how to make an inverse function graph.
What Is the Inverse Function?
An inverse is a function that does the opposite of what another function does. If a function is invertible, it means it can be changed.
An inverse function is written as f-1. It’s best to show inverses with an arrow diagram, like this one:
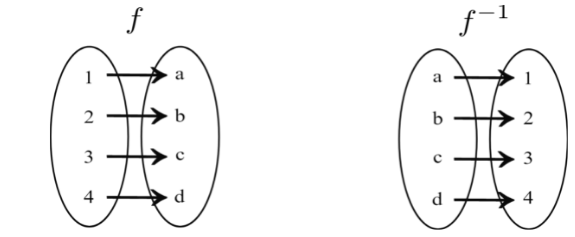
When you look at how f maps ‘1’ to ‘a’, f-1 does the opposite of f. It maps ‘a’ back to 1.
Most things have opposites, but not all of them do. The opposite of something never has the same value again, so it’s easy to figure out.
It is one-to-one when f(x) is one-to-one. For one-to-one properties, we use the Horizontal Line Test (HLT) for functions.
How to Graph the Inverse of a Function
Both the function and its inverse are shown on the line that goes from y to x, which shows both. A line goes through the origin and has a slope of 1.
When you’re asked to draw a function and its inverse, a dotted line is one of the ways you can do it. So, you can see the points of the function change as they move across the line. You can also see them in real-time as they move across it. You can figure out the inverse without writing down a lot of points by reversing that line so that you can do that.
When you can see this concept in action, you’ll be able to understand better what it is about. Let’s say you already know that these two things are inverse to each other.
f(x)=3x-2
g(x)=(x+2)/3
Do these things:
- Make up a number (any number you want) and put it into the function that comes first.
- F(-4) is -14. It says (-4,-14) in writing,
- In Step 1, you used that value to do something else. Find g(-14) next. That gives you -4.This shows they are inverse of each other.
The point (a, b) turns into the point (b, a) in its other direction. You first need to figure out how the function looks. Then, change the values of each point in each point so that you can see how the opposite looks. Make sure you look at the line “y = x” and see how the values move from one function to the next.
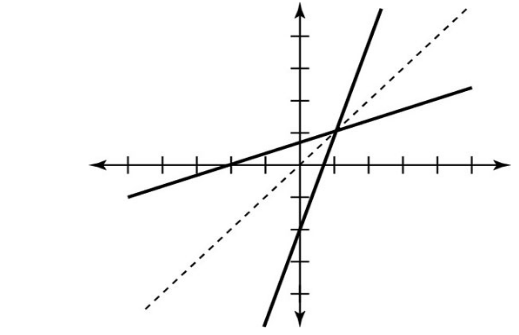
f(x) = 3x -2 is no longer important. To graph the inverse function, you can use the slope-intercept form.
- To begin, graph y = x. There is a slope-intercept form that tells you where the y-intercept is so that you can find it.
- (0, –3): It takes you 3 units up and 1 unit over to get to the point (1, 1).
- You reach the point by moving three units and one unit again (2, 4).
- This way, you can see how the inverse function moves through (–2, 0), (1, 1), and (–2, 0) before it comes to an end (4, 2).
- In this picture, you can see both the function and the opposite of that function.
How to Undo a Function: Example
Example: f(x) = X2.
f(2) and f(-2) both go to 4.
In f-1(x) putting x=4 will give two values of x hence f-1(x) is not a function.But we can make it a function by specifying certain boundaries for example:
f(x) = X2 X≥0.
In this case, f(2) = 4
The inverse of f would take 4 back to 2.
Thus, the inverse of f would have to take 4 to 2, which will make it the correct inverse.
There are ways to look at the same thing in a graph. If f had an opposite, its graph would look like f’s graph about the line y = x.
Conclusion
If a function does something, the inverse function does the same thing backwards. In this section, we covered the definition of an inverse function.
We also look at the relationship between the graph of a function and its inverse graph. Then, we use these ideas to talk about the properties of the inverse trigonometric functions, like how they work and what they look like.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out




