Profile
Settings
Refer your friends
Sign out
When two straight lines or line segments intersect with each other, then four angles are formed. These angles are a pair of two different angles. The different criteria on which different angles are categorized are:
On the basis of their value
On the basis of their position
On the basis of their sum
When two lines or line segments cross each other in a plane, they are called intersecting lines. The point at which these lines or line segments intersect is called the point of intersection.
Types of angles formed when two lines intersect
When the categorization of angles is done based on their value, the following angles are formed.
Acute angle
Acute angles are the angles formed that have a value less than 90 degrees.
Obtuse angle
Obtuse angles are the angles formed that are more than 90 degrees and less than 180 degrees in value.
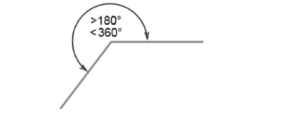
Reflex angle
Reflex angles are the angles formed by two intersecting lines whose value is more than 180 degrees and less than 360 degrees.
Right angle
Right angles are the angles formed by two lines that are intersecting at exactly 90 degrees in value.
Straight Angle
When the measure of the angle formed by two intersecting lines is 180°, it is known as a straight angle.
When the categorization of angles is done on the basis of their position, the following angles are formed.
Adjacent Angles:
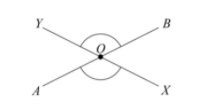
The angles formed next to each other are known as adjacent angles. These angles may or may not be equal. When two lines or line segments intersect each other, four pairs of adjacent angles are formed.
Here, the four pairs of adjacent angles are
∠AOY and ∠AOX
∠AOY and ∠YOB
∠YOB and ∠BOX
∠BOX and ∠XOA
Vertically Opposite Angles:
When two straight lines intersect with each other, opposite angles are also formed. There are two pairs of vertically opposite angles formed. Opposite angles are equal in value. They are also called vertical angles. Vertical itself means equal.
In the above image,
∠AOY is vertically opposite to ∠XOB
Likewise,
∠BOY is vertically opposite to ∠XOA
Linear Pair of Angles
When the adjacent angles are formed on a straight line, then the angles formed are known as linear pairs, and their sum is always 180°.
When the categorization of angles is done on the basis of the sum of the angles, the following angles are formed.
Complementary Angles
The angles whose sum is 90° are known as complementary angles. For example, if we have ∠A = 60° and ∠B = 30°
∠A + ∠B = 60° + 30° = 90°
Therefore, they are complementary angles.
Supplementary Angles
The angles whose sum is 180° are known as supplementary angles. For example, if we have ∠A = 120° and ∠B = 60°
∠A + ∠B = 120° + 60° = 180°
Therefore, they are supplementary angles.
Conclusion
Angles are formed as a result of the intersection of two lines with each other. When it comes to the intersection of lines, straight lines can only cross each other at one particular point. This point where two straight lines intersect each other is called the point of intersection. It is at this point of intersection where different angles are formed. The angles that are next to each other are known as adjacent angles. These angles can be supplementary, and their sum is always equal to 180 degrees. Whereas, the angles that are opposite to each other are called opposite angles. Opposite angles are equal in value and are also called vertically opposite angles.
