Profile
Settings
Refer your friends
Sign out
What is Trigonometry?
The word ‘trigonometry’ is derived from the Greek words ‘trigon’ and ‘metron’, and it means ‘measuring the sides of a triangle. The subject was originally developed to solve geometric problems involving triangles. Trigonometry is an essential section of mathematics; trigonometric functions and identities are used in solving most mathematical problems. If students don’t have a clear understanding of this chapter, they will face trouble in solving questions of other chapters too.
Trigonometry Functions
Trigonometry functions, also called circular functions, are defined as the relationship between the angles of a triangle with the triangle.
Basic trigonometric functions, also called trigonometric ratios are sine(sin), cosine(cos), cosecant(cosec), tangent(tan), secant(sec), and cotangent(cosec). various trigonometry identities and formulas that are formed using these 6 trigonometric ratios which establish the relationship between the trigonometric functions and allow them to find the angle of the triangle which is not known.
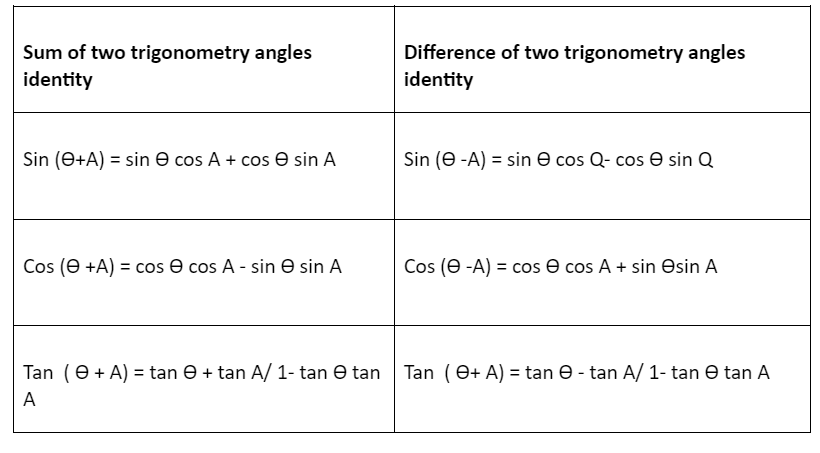
Trigonometric Functions of Sum and Difference of 2 Angles
Sum and difference of angles is an important aspect in trigonometry if you want to solve problems like measuring the height of a mountain or calculating larger distances like the distance between the sun and the earth. The sum and difference of angles in trigonometric functions are trigonometric identities. The trigonometric identities of sum and difference of angles are also helpful in simplifying many trigonometric equations and expressions.
Two trigonometric expressions:
The following equations in trigonometry will be used in this article to establish the relation between sum and difference of angles in trigonometry functions
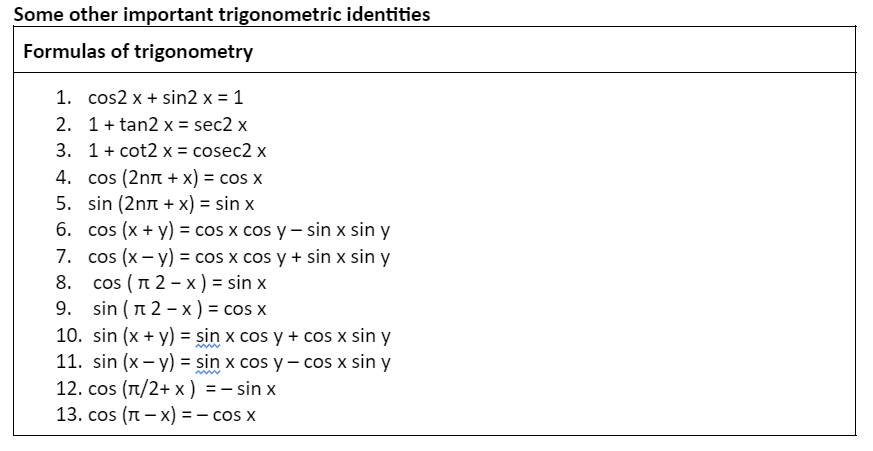
cos(-x) = cosx
Sin (-x) = -sinx
Trigonometry Functions
There are multiple trigonometry formulas and identities which represent the relation between the functions and enable them to find the unknown angle of a triangle.
Sum and Difference of 2 Angles Formulas
Some Solved Examples of Trigonometric Functions of Sum and Difference of 2 Angles
- Apply trignometric identities and convert each of the following products into the sum or difference of angles.
(i) 2 sin 40° cos 30°
(ii) 2 sin 75° sin 15°
(iii) cos 75° cos 15°
Answer:
(i) Given: A = 40° and B = 30°
Now put the given values in the formula above learned,
2 sin A cos B = sin (A + B) + sin (A – B)
We get
2 sin 40° cos 30° = sin (40 + 30) + sin (40 – 30)
= sin (70°) + sin (10°)
(ii) Given: A = 75° and B = 15°
Now put the given values in the formula above learned,
2 sin A sin B = cos (A – B) – cos (A + B)
We get
2 sin 75° sin 15° = cos (75-15) – cos (75+15)
= cos (60°) – cos (90°)
(iii) Given: A = 75° and B = 15°
Now put the given values in the formula above learned,
2 cos A cos B = cos (A + B) + cos (A – B)
We get
cos 75° cos 15° = 1/2(cos (75+15) + cos (75-15))
= 1/2 (cos (90°) + cos (60°))
Conclusion
The sum and difference of angles in trigonometric functions are trigonometric identities. The trigonometric identities of sum and difference of angles are also helpful in simplifying many trigonometric equations and expressions.
