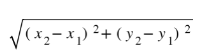Distance formula in 3D system

In the distance formula, we usually find the distance between two points on a plane. The distance formula tends to be used when you know the coordinates on the points you need to substitute the formula to find the two points on a given plane. To locate the location of a given point on a plane surface you need to have a coordinate axis. The distance from the middle i.e the center is the x-axis and the point taken from a distance is  the y-axis.

What is the distance formula in the 2D coordinate system?

The distance formula in the 2D coordinate system for any two points (x1,y1) and (x2,y2) is given as: 

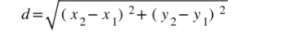
The trick of finding two points in Distance Formula: 2D Coordinate System?

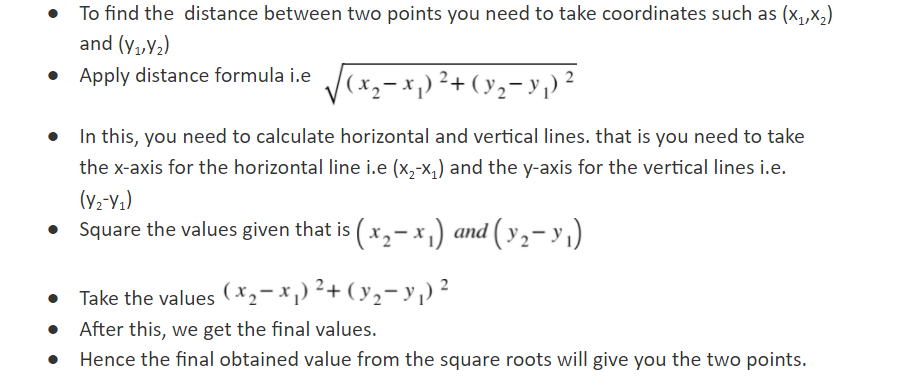
To find the distance of the points  on the plane here are the following steps you need to follow.

The distance formula between two points is in 3D coordinate system is given as: 

Let the 2 points be P(x1,y1,z1) and Q(x2,y2,z2)

As ∠PAQ forms a right angle,

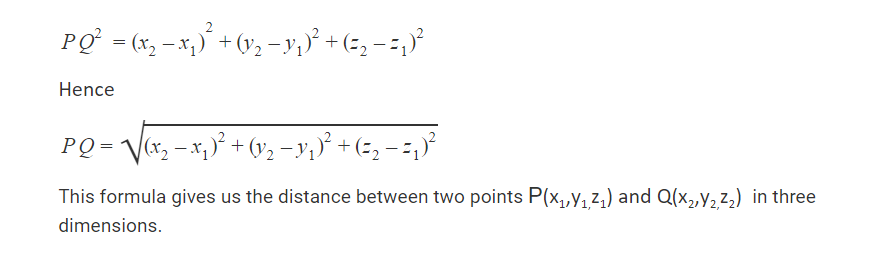
Examples

Example 1:

Find the distance between the two points given by P(5, 3, -3) and Q(1, -9, 3).

Example 2 The distance between the points (16, 5) and (12, 2) is equal to

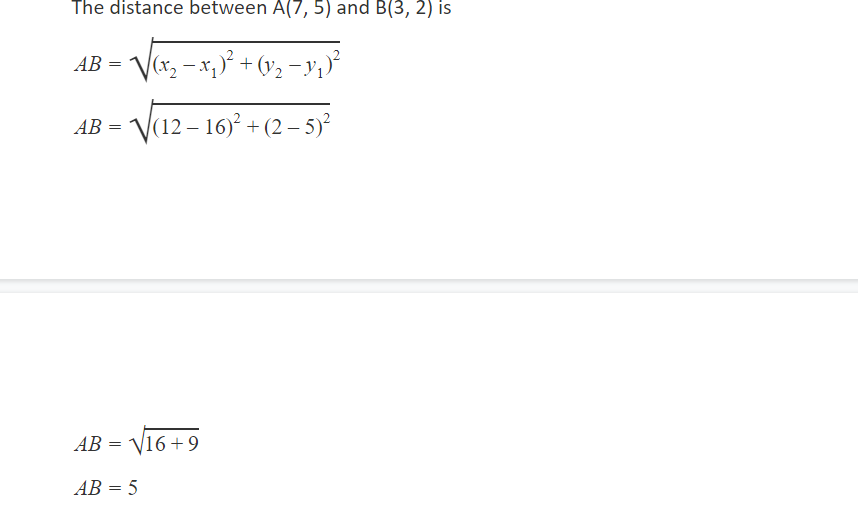
Example 3: Obtain the distance between points A (4, 17) and B (10, 9).

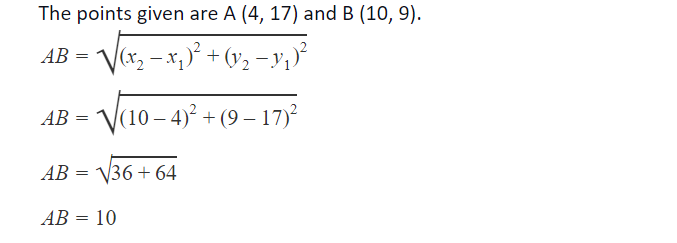
Example 4: If the distance between the points (a, 2) and (3, 4) be 8, then a =

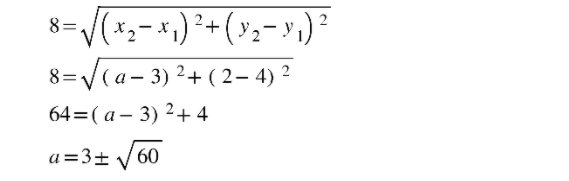
Example 5: Compute the distance between the two points (2, – 9) and (7, 3).

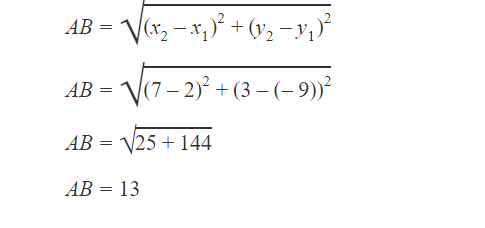
Conclusion

To find the distance between two 3D coordinates is 

The distance formula tends to be used when you know the coordinates on the points you need to substitute the formula to find the two points on a given plane. To locate the location of a given point on a plane surface you need to have a coordinate axis. You can also use this formula as a distance formula calculator.