Algebra of Limits

Limits are the values that are further used to define continuity, derivatives, and integrals.

Generally, the limit of a function is written as:

xcf(x)=L

Here x→c denotes the value of x that is tending to c.

Further, the function’s value at the points to the left of a point ‘c’ is the left-hand limit of the function at that point. It is denoted as lim x→c−. This is equal to the value shown by it at the rightward of a point ‘c’, which shows the value of the function. This is the right-hand limit of the function at that point and is denoted as lim x→c+. 

Here the function is defined only when the right-hand limit is equal to the left-hand limit of the function.

The solution of sets of equations is an essential and very beneficial part of algebra. Algebra is commonly used in formulas when we can change one of the numbers or at least one of the numbers is unknown. This article teaches you about the algebra of limits.

Algebra methods are also used to evaluate the limits. Some of the important methods are factorisation method, evaluation using standard limits, direct substitution method, rationalisation and evaluation of limits at infinity but before that, let’s learn about a few algebraic properties of limits.

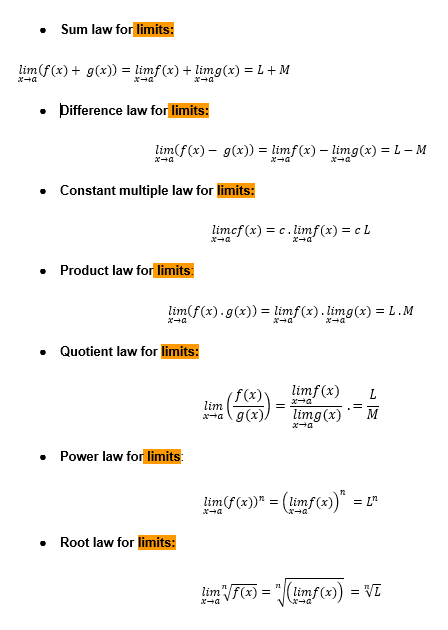
PROPERTIES OF LIMITS:

Let’s now dive into few algebraic properties of limits:

ALGEBRAIC METHODS FOR EVALUATION OF LIMITS:

1. Plugging the value of x:

The first technique for solving a limit algebraically is to plug the number x into the function. If you get an undefined value (0 in the denominator), you must move on to another technique. But if your function is continuous at that x value, you will get a deal, and you’re done; you’ve found your limit!

1. Factoring:

Factoring is the method to try when plugging fails, especially when any part of the given function is a polynomial expression. Factoring is beneficial before and after, many times after simplifying the equations. This method generally involves making factors of polynomials in the numerator and denominator of the equation. Then, we cancel the common values typically out of elements and plug the value of limits in the rest of the equation. 

1. Rationalising the numerator:

The third technique you need to know to find limits algebraically requires rationalising the numerator. Functions that require this method have a square root in the numerator and a polynomial expression in the denominator. Plugging in numbers fails when you get 0 in the fraction’s denominator. Factoring fails because the equation has no polynomial to factor.

1. Finding the lowest common denominator:

When given a complex rational function, you use the fourth and final algebraic limit-finding technique. The plugging approach fails because you end up with a 0 in one of the denominators. The function isn’t factorable, and you have no square roots to rationalise. You combine the tasks by finding the least common denominator (LCD). The terms cancel, at which point you can find the limit.

1. SANDWICH THEOREM:

The Sandwich Theorem or squeeze theorem is used for calculating the limits of given trigonometric functions. This theorem is also known as the pinching theorem. This theorem is generally used in calculus, including mathematical analysis. This theorem is probably used to establish the limit of a function by comparing two other functions whose limits are known or surely figured.

SOME STANDARD LIMITS:

x0sin x=0

x0cos x=1

x0sinx/x=1

x0log(1+x)/x=1

x0logex=1

xelogex=1

x0logex−1/x=1

x0logax−1/x=logea

CONCLUSION:

Algebraic expressions are used in almost every sect of mathematics; solving these expressions has different methods and ways for various topics in maths. Limits are essential to learning before moving to core calculus and mathematical analysis. Similarly, limits also use algebra to simplify the equations and get valid output. Some basic methods are factorisation, evaluation using standard limits, direct substitution, rationalisation, and assessment of limits at infinity.