Logarithm Types

A logarithm is a term and concept coined by Scottish mathematician John Napier. A logarithm is formed by combining two Greek words: logos, which means principle or thought, and arithmos, which means number.

A logarithm is a number that must be multiplied by a given amount. The log of a number is used to represent it. For instance, log (x). Logarithm If y = a^x then log_ay= x   a is the base. The exponent is x. where a>0, a1, and y⁰ For instance: 2⁵ = 32 

Log₂32= 5

Logarithmic properties

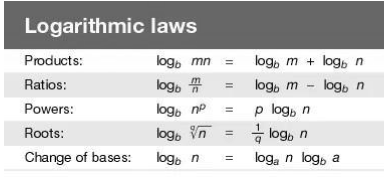
Scientists immediately adopted logarithms due to a variety of advantageous qualities that made long, painstaking calculations easier. Scientists may, for example, find the product of two numbers m and n by looking up the logarithms of each number in a special table, adding the logarithms together, and then consulting the table again to find the number with the calculated logarithm (known as its antilogarithm). 

This relationship is expressed in terms of common logarithms as log mn = log m + log n. 100 1,000, for example, can be determined by looking up the logarithms of 100 (2) and 1,000 (3), putting the logarithms together (5), and then looking up the antilogarithm (100,000) in the table. Similarly, logarithms are used to convert division difficulties into subtraction problems: log m/n = log m log n.

 Not only that, but logarithms can be used to simplify the calculation of powers and roots. As illustrated below, logarithms can be translated between any positive bases (excluding 1, which cannot be used as the base because all of its powers are equal to 1)

What Are The Different Types Of Logarithms?

Logarithms are divided into two types:

• The base 10 logarithm is the most common logarithm. The symbol for it is log₁₀.

• The base e logarithm is a type of natural logarithm. The symbol for it is log_e.

Common logarithm

The logarithm with base 10 is known as the common logarithm in mathematics. It’s also known as the decadic logarithm, decimal logarithm, and Briggsian logarithm, after Henry Briggs, an English mathematician who pioneered its application.

It was previously referred to as logarithmus decimalis or logarithmus decadis. It’s written as log(x), log10 (x), or occasionally Log(x) with a capital L. (however, this notation is ambiguous, since it can also mean the complex natural logarithmic multi-valued function). It’s written as “log” on calculators, but when mathematicians say “log,” they usually mean natural logarithm (logarithm with base e 2.71828) rather than common logarithm.

 To avoid ambiguity, the ISO 80000 standard proposes that log₁₀(x) be written log(x) and log_e (x) be written ln (x).

The Natural Logarithm: An Introduction (ln)

The natural log isn’t exactly “natural” in the way it’s portrayed in textbooks: it’s defined as the inverse of, which is already a peculiar exponent.

But there’s a new, intuitive explanation: the natural log tells you how long it will take to reach a specific degree of development.

growth vs. natural log time

Assume you have a gummy bear investment (who doesn’t?) that pays a 100% annual interest rate and continues to increase. If you want 10x growth, you’ll have to wait only 2.302 years assuming continued compounding. Why does it take only a few years to achieve 10x growth? Why is it that the pattern isn’t 1, 2, 4, 8? Find out more about e.

The Natural Log and e are twins:

• e is the amount we have after starting at 1.0 and growing constantly for units of time, and 

• ln(x) (Natural Logarithm) is the time it took us to achieve amount if we grew continuously from 1.0.

Isn’t it reasonable? Let’s delve into the intuitive explanation while the mathematicians scurry to give you the long, technical explanation.

E stands for expansion

The letter e stands for endless expansion. Let us combine rate and duration, as we observed last time: 3 years of 100% growth is the same as 1 year of 300% growth when compounded constantly.

For simplicity, we can convert any rate and period combination (50 percent for four years) to 100 percent (giving us 100 percent for 2 years). We simply need to consider the time component when converting to a rate of 100%:

Intuitively, this implies:

After x units of time, how much growth do I get? (and 100 percent continuous growth)

For example, I have e³ = 20.08 times the amount of “things” after three time periods.

e^x is a scaling factor that tells us how much growth we’ll obtain after a certain number of time units.

It’s All About Time in Natural Log

The inverse of is the natural log, which is a fancy word for opposite. The Latin term for this creature is logarithmus naturali, which is abbreviated as ln.

So, what exactly does inverse or opposite mean?

Let’s plug in some time and see what happens.

Let’s plug in some growth and see how long it will take.

For instance:

• e³ is 20.08. We finish up with 20.08 times what we started with after three units of time.

• ln(x) is about 3. We’d have to wait three units of time for growth of 20.08. (again, assuming a 100 percent continuous growth rate).

With me? The natural log provides us with the necessary time to achieve our desired growth.

Conclusion

A logarithm is a term and concept coined by Scottish mathematician John Napier. A logarithm is formed by combining two Greek words: logos, which means principle or thought, and arithmos, which means number.

The natural log isn’t exactly “natural” in the way it’s portrayed in textbooks: it’s defined as the inverse of, which is already a peculiar exponent.

But there’s a new, intuitive explanation: the natural log tells you how long it will take to reach a specific degree of development.

The logarithm with base 10 is known as the common logarithm in mathematics. It’s also known as the decadic logarithm, decimal logarithm, and Briggsian logarithm, after Henry Briggs, an English mathematician who pioneered its application.