The Carius halogen technique is a quantitative method for determining halogens in chemical compounds in analytical chemistry.
In a furnace, a fixed quantity of an organic compound is heated in the mere presence of silver nitrate placed in a hard glass tube called a carius tube. The compound’s carbon and hydrogen are oxidised to carbon dioxide and water. The presence of halogen results in the formation of silver halide (AgX). It is filtered, rinsed, dried, and weighed before being used.
This chemical test is as effective for determining sulphur levels without the use of silver nitrate. With the addition of barium chloride, the sulphuric acid intermediate generated by the interaction of sulphur with fuming nitric acid transforms into insoluble barium sulphate. The addition of nitric acid serves to oxidise carbon and hydrogen. Nitric acid in concentrated form solely oxidises iodine to iodic acid and has no effect on other halogens. Iodine oxidation by concentrated nitric acid occurs only at extremely high temperatures.
Quantitative analysis
Quantitative analysis is a type of analysis that may be used to figure out how many elements or molecules are created during a reaction. Carbon and hydrogen make up organic molecules. Oxygen, nitrogen, phosphorus, sulphur, and halogens are among the elements found in them.
Quantitative Analysis of Halogens
Carius Method
In this experiment, we heat an organic molecule with fuming nitric acid in the presence of silver nitrate in a tube (hard glass). In a furnace, this tube is known as the Carius tube. The organic compound’s carbon and hydrogen oxidised to carbon dioxide and water, respectively.
The organic compound’s halogens react with the silver nitrate to generate the matching silver halide (AgX). We filter, wash, dry, and weigh them after that.
Calculations
Let us decide the mass of the given organic compound as g. Suppose the total mass of the compound, AgX formed . We know that AgX has 1 mol of X. So, in of AgX,
Mass of halogen = (atomic mass of X x m1g) molecular mass of AgX)
Percentage of halogen = ( atomic mass of X x m1 x 100) (molecular mass of Ag X x m )
How Does the Carius Method Estimate Sulphur?
In the Carius technique, a known quantity of sulphur-containing organic compound is heated in a sealed Carius tube in the presence of excess fuming nitric acid. This organic compound contains sulphur, which is transformed to sulphuric acid. An excess of barium chloride solution is used to treat this sulphuric acid. The mineral barium sulphate is produced and precipitated. Afterwards, the material is filtered, washed, dried, and weighed. The sulphur is determined by this proportion. As a result, this procedure is known as the Carius technique of sulphur estimation.
Solved Example:
In Carius’ method of estimation of halogens, 0.15 g of an organic compound gave 0.12g of AgBr. What is the percentage of bromine in the compound?
Hint: The Carius technique is used to determine the presence of halogens in organic compounds. This approach is used in analytical chemistry and is a quantitative analysis. By using this approach, we may estimate the proportion of halogens using a formula. The formula is as follows:

Complete Step by Step Solution
We usually utilise the Carius halogen technique to figure out how many halogens are in a chemical molecule.
In this procedure, we heat a known mass of an organic compound in a hard glass tube with fuming nitric acid in the presence of silver nitrate. The carbon and hydrogen in the compound are oxidised to generate carbon dioxide and water, and the halogen compound reacts with the silver nitrate to form a silver halide precipitate, which is dried and weighed and used to calculate the halogen quantitatively.
Here, the weight of the organic compound
Weight of the silver bromide precipitate
We know that, atomic mass of bromine
Atomic mass of silver bromide
Therefore, according to the Carius method, we can write that-
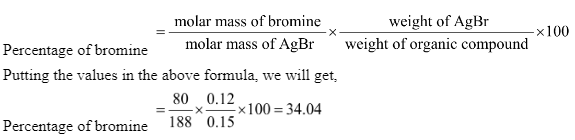
Therefore, the correct answer is 34.04%.
Note: In this procedure, nitric acid is used to convert carbon and hydrogen into carbon dioxide, which will then develop into gas and water, which may be eliminated when heated. Any halogen is unaffected by nitric acid. However, concentrated nitric acid may oxidise iodine to iodic acid, but this requires a very high temperature.
Conclusion
The Carius technique involves heating an organic compound in a sealed tube with silver nitrate in strong nitric acid to determine the quantity of sulphur and halogens present. Silver sulphide and halides are precipitated, separated, and weighed after the complex is decomposed. The Carius technique is used to calculate halogen.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out








