Introduction
Trigonometry has a wide range of applications, mostly in engineering, architecture, astronomy, and navigation. One of them is to measure height and distance between two objects or places by applying different formulae. These formulae help in finding out the accurate height, distance, depth, and angle between different objects.
Using trigonometry, astronomers and scientists have even found the distance between the Earth and the Sun. Using the height and distance formulae and ratios, we can even find out the height of the highest mountains. This article explains how all these mathematical techniques help in finding out height and distance without actually measuring them.
Line of Sight
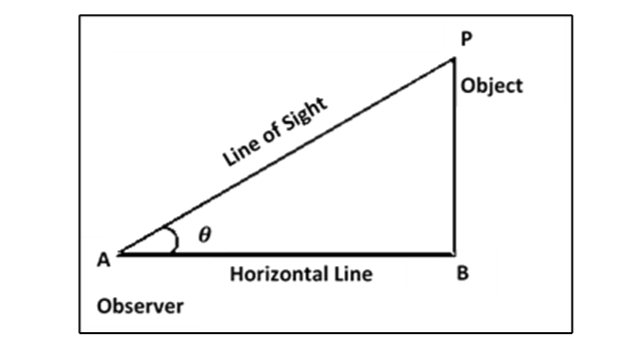
Angle of Elevation
As shown in the above figure, when an observer looks at the object (P), at a greater height than (A), the person has to look at the object by lifting his/her eyes to see the object.
An angle PAB is formed by the line of sight and the corresponding line AB. This is the angle of elevation. In other words, when the object is above the eyesight of the person, it forms the angle of elevation. In short, when the line of sight is above the horizontal line AB, it forms the angle of elevation. For instance, when the person raises his or her head to look up at the object, the angle of elevation is formed.
Angle of Depression

The angle of depression, on the other hand, happens when an observer (A), who is at a greater height, looks at an object that is of a lesser height. In simple words, when the person lowers his or her head to look at the object, it forms the angle of depression. Hence, it can be said that the angle of depression is the exact opposite of the angle of elevation.
Height and Distance
After understanding the line of sight, angle of elevation, and angle of depression, the calculation of the height and distance of an object becomes easier. To calculate the height and distance of a given object, it is necessary to know the distance at which the person is standing from the foot of the object, the height of the person, and the measurement of the angle of elevation or the angle of depression.
Here, we can consider figure (a) and assume that we already know all the values. Now, to determine the height and distance of the tower, we need to use the appropriate trigonometry ratios.
In the figure (a), line CD = line CB + line BD and line BD = line AE. To find out the line BC, we can use trigonometry ratios, considering the angle CAB. Here, the line BC is in the opposite direction of the angle CAB. Hence, we can either use tan A or cot A. By using tan A, we get,
tan A = BC/ AB = BC,
Therefore, by adding BC and AE, we will determine the height of the tower.
Formulae
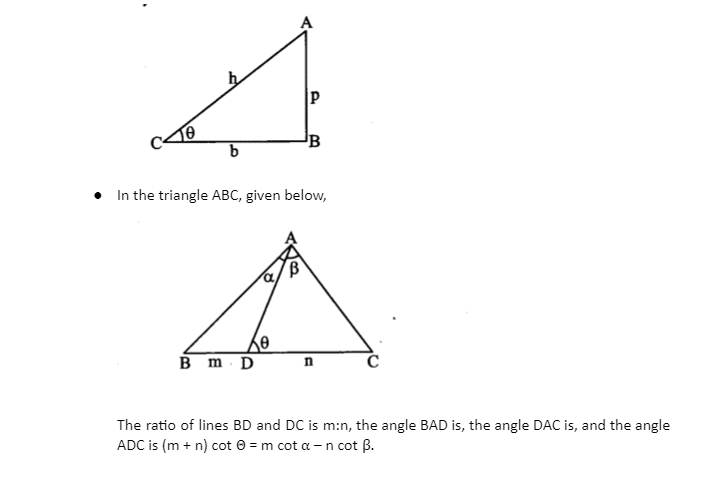
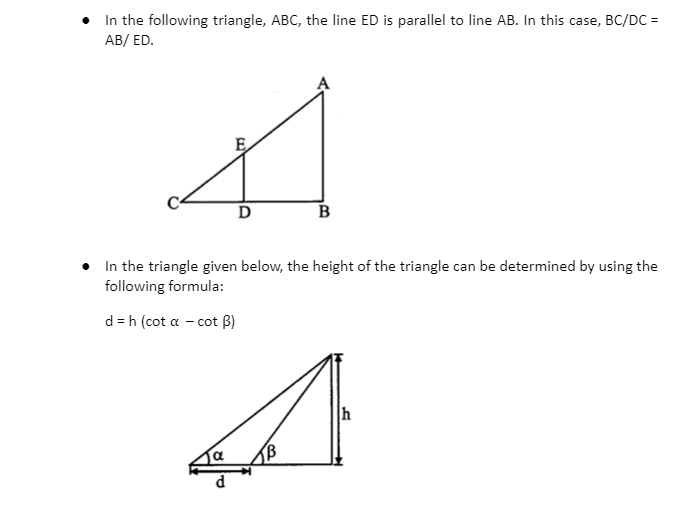
- In the below-given triangle, ABC, we can use the following trigonometric ratios to determine the height of the triangle, that is, the line AB.
Important points to note while solving height and distance problems:
- Sometimes, the point of the observer is given instead of the height of the observer
- If the height or length is not given, we can consider the line segment or the respective point of the given object
- Remember to consider the line parallel to the earth’s surface as the horizontal line
- Both the angles of elevation and the angles of depression are always acute
- In the case of the angle of elevation, the height of the object increases as the person moves towards it and vice versa
- When the angle of elevation of the sun decreases, the shadow of the respective object increases, and the same goes the other way round
Conclusion
The height and distance between two or more objects are determined based on different trigonometric ratios. Along with this, we need to find out the line of sight to determine whether the angle of elevation or angle of depression is formed. Moreover, certain other factors such as the height of the observer and the measurement of the angles are necessary to find out the height and distance of the respective object. Here, the line of sight means the line that is formed from the point of the eyesight of the observer to the point at the top of the object. The angles of elevation and depression are determined based on the horizontal line formed parallel to the ground.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out




